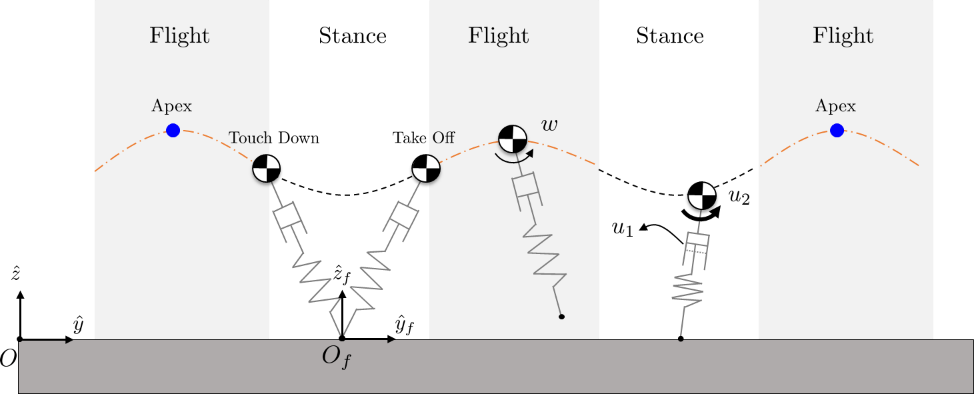
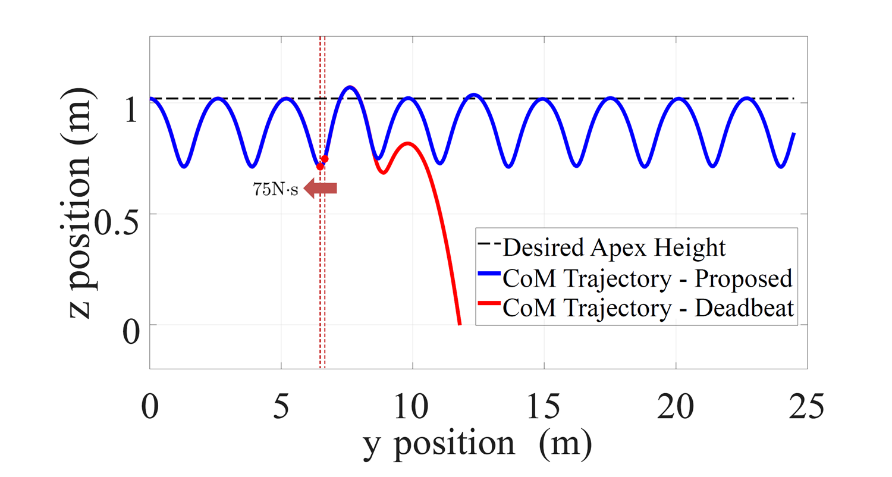
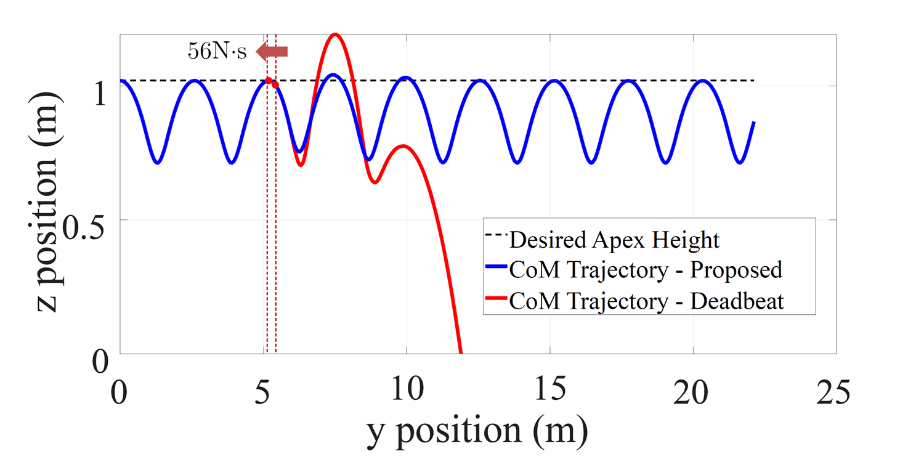
Our new paper entitled “Optimal Control of a Differentially Flat 2D Spring-Loaded Inverted Pendulum Model” is accepted by IEEE Robotics and Automation Letters (RA-L). We study the optimal control problem of an extended spring-loaded inverted pendulum (SLIP) model with two additional actuators for active leg length and hip torque modulation. These additional features arise naturally in practice, allowing for consideration of swing leg kinematics during flight and active control over stance dynamics. Nonlinearity and the hybrid nature of the overall SLIP dynamics introduce challenges in the analysis and control of the model. We first show that the stance dynamics of the considered SLIP model are differentially flat, which has a strong implication regarding controllability of the stance dynamics. Leveraging this powerful property, a tractable optimal control strategy is developed. This strategy enables online solution while also treating the hybrid nature of the SLIP dynamics. Together with the optimal control strategy, the extended SLIP model grants active disturbance rejection capability at any point during the gait. Performance of the proposed control strategy is demonstrated via numerical tests and shows significant advantage over existing methods. This is a joint work with Prof. Patrick M. Wensing at University of Notre Dame. The paper can be found here (https://arxiv.org/abs/1911.07168).